y=f(x) will indicate that the graph has reflected about the origin If I have f(x)=(x) 3 , then the graph would be reflected about the yaxis Try to experiment on these transformations by using a graphing utility such as Desmos , Symbolab , GeoGebraGraph transformation is the process by which an existing graph, or graphed equation, is modified to produce a variation of the proceeding graph It's a common type of problem in algebra, specifically the modification of algebraic equations Sometimes graphs are translated, or moved about the xObjective Demonstrate mastery of function transformations algebraically and graphically Priority Standard MGSE912FBF3 Identify the effect on the graph of replacing f(x) by f(x) k, k f(x), f(kx), and f(x k) for specific values of k (both positive and negative);
Q Tbn And9gcsl0jyluxfxjjcizs7jyiyflsxbm6hpbpzdawckl4kjhmo0pmir Usqp Cau
1/f(x) graph transformation
1/f(x) graph transformation-Answer to Describe transformation from the parent graph f(x) = x to the graph of each of the following functions f (x) = 1x21 f(x) = 1x51 f(x) =The transformations you have seen in the past can also be used to move and resize graphs of functions We will be examining the following changes to f (x) f (x), f (x), f (x) k, f (x k), kf (x), f (kx) reflections translations dilations



Transformations Of Functions Mathbitsnotebook A1 Ccss Math
Algebra Q&A Library Describe verbally the transformations that can be used to obtain the graph of g from the graph of f g(x) = 9* 6, f(x) = 9* Select the correct choice below and, if necessary, fill in the answer box(es) within your choice (Type integers or simplified fractions) O A The graph of g is the graph of f reflected in the xaxis O B The graph of g is the graph of f shiftedReflections and stretches/compressions have first priority and can be done in any orderSection 56 Graphical Transformations In this section we are going to explore the graphical repercussions of alterations to a given function formula Specifically, we are going to explore how the graph of the function \(g\) compares to the graph \(f\) where
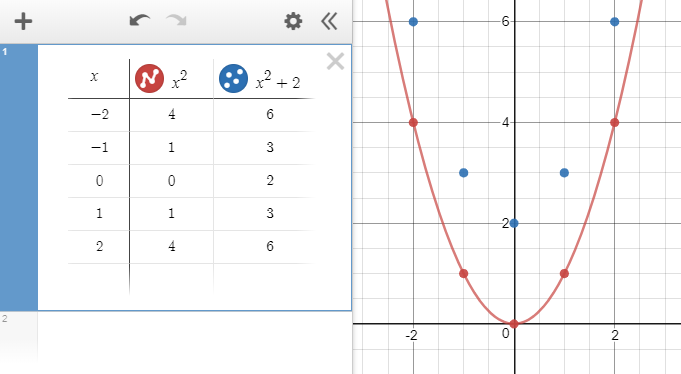
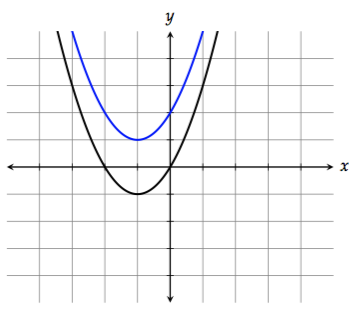
Transformations of functions mean transforming the function from one form to another Transformation of functions is a unique way of changing the formula of a function minimally and playing around with the graph Look at the graph of the function f (x) = x2 3 f ( x) = x 2 3 Did you observe that the graph is 3 units above the quadraticGet stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us! 17 Transformations In this section, we study how the graphs of functions change, or transform, when certain specialized modifications are made to their formulas The transformations we will study fall into three broad categories shifts, reflections and scalings, and we will present them in that order
AnswerA right 2, up 3Stepbystep explanationWe have that,The function is transformed to We see that,The function f(x) is translated 2 units to the right and 3 units upwards to obtain the function g(x)So, the correct transformation is 'right 2, up 3'Hence, option A is correctFunctions of graphs can be transformed to show shifts and reflections Graphic designers and 3D modellers use transformations of graphs to design objects and imagesDescribe the transformation of the graph of f(x) to f(−x) Reflect across y axis (all x values change sign) What is the correct order of vertical transformations?



Desmos 2 Transformations Of Graphs Cambridge Maths Hub




The Function F X Is Shown On The Provided Graph Graph The Result Of The Following Transformation On Brainly Com
Let's examine it more closelyF(x) k Shifts a graph up k units Add k units to y f(x) – k Shifts a graph down k units Subtract k units from y Reflections cause a graph to rotate (or flip) over the xaxis or yaxis Transformation What will happen? Which transformation of y=x^3 needs to be applied to graph f(x) = (x 3)^3 4 Which transformation of y=x^3 needs to be applied to graph f(x) = (x 3)^3 4 Categories Uncategorized Leave a Reply Cancel reply Your email address will not be published Required fields are marked *



Graphing Y F 2x As A Transformation Of Y F X Math Showme




Transforming Graphs Of Functions Brilliant Math Science Wiki
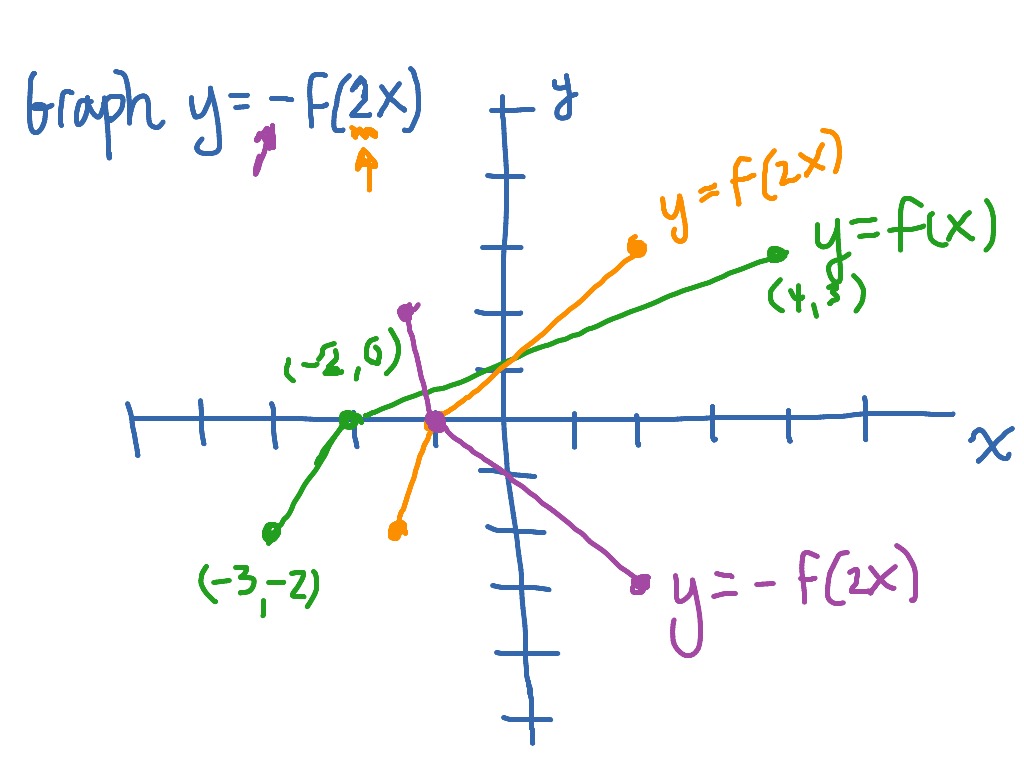
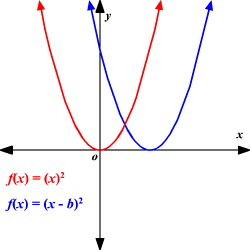
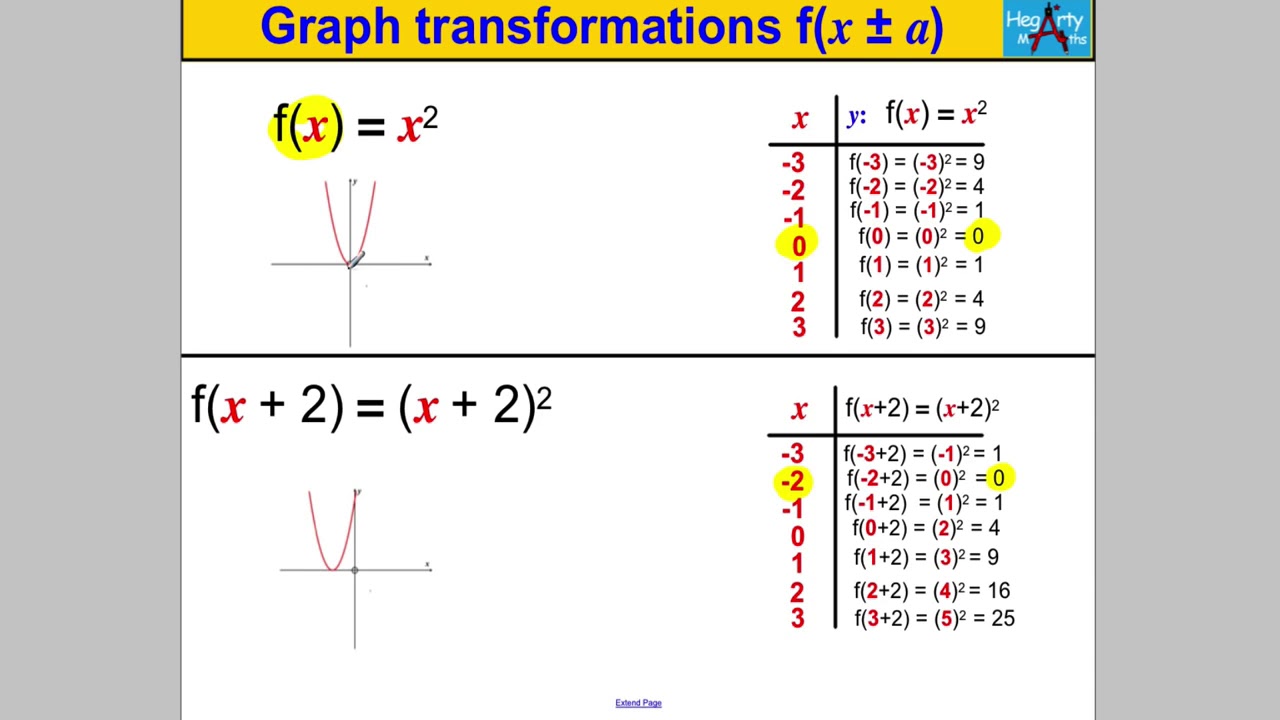
– f(x) Reflects a graph across the xaxis Take the opposite of each y f(– xF(x) just describes any function it could be a quadratic, a linear graph, a circle or any other function For example, here is a random function If we apply the transformation y = af(x), we are multiplying every value of the function by a (which could17 Transformations 123 2 to all of the xvalues of the points on the graph of y= f(x) to e ect a shift to the right 2 units Generalizing these notions produces the following result




Transforming Graphs Of Functions Brilliant Math Science Wiki



How To Combine Various Transformations Dummies
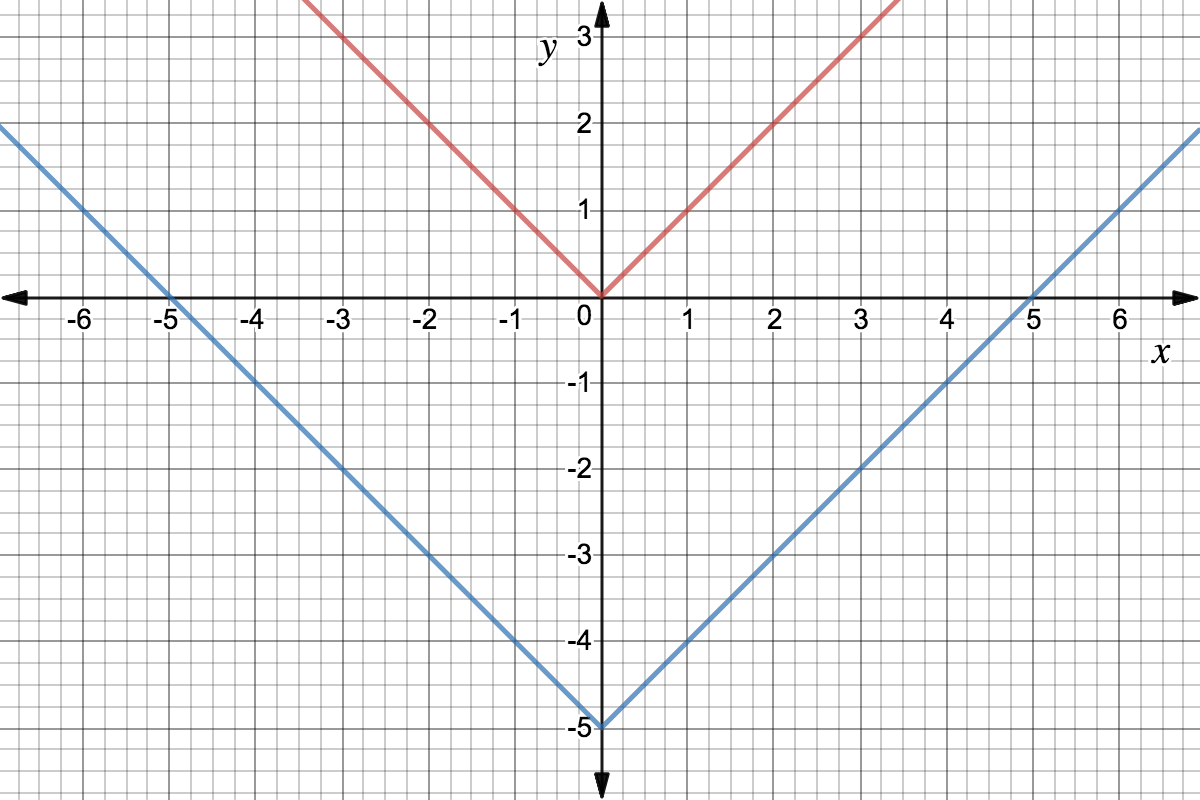
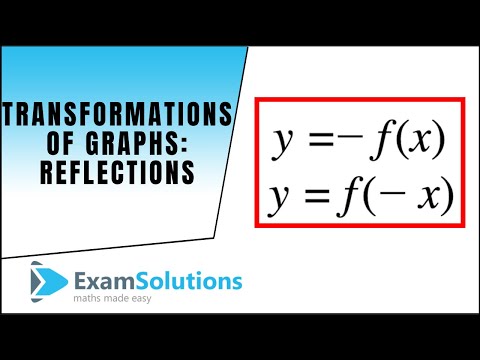
Example 2 Write the steps to obtain the graph of the function y = 3 (x − 1) 2 5 from the graph y = x 2 Solution Step 1 By graphing the curve y = x 2, we get a open upward parabola with vertex (0, 0) Step 2 Here 1 is subtracted from x, so we have to shift the graph of y = xGraph f (x)=x Find the absolute value vertex In this case, the vertex for is Tap for more steps To find the coordinate of the vertex, set the inside of the absolute value equal to In this case, Replace the variable with in the expression The absolute value is the distance between a number and zero The distance between and isSection 36 Transformations of Graphs of Linear Functions 147 CCore ore CConceptoncept Monitoring Progress Refl ections in the xaxis The graph of y = −f(x) is a refl ection in the xaxis of the graph of y = f(x) x y y = −f(x) y = f(x) Multiplying the outputs by −1 changes their signs Refl ections in the yaxis The graph of y = f(−x) is a refl ection in the yaxis of the graph of y




How To Graph Transformations Of Functions 14 Steps
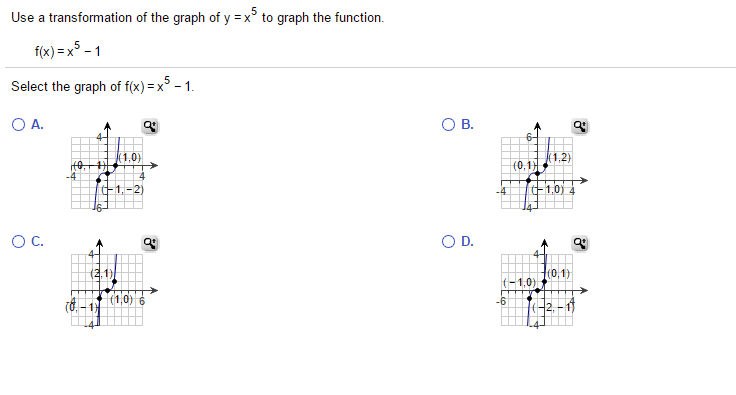



Use A Transformation Of The Graph Of Y X 5 To Graph Chegg Com
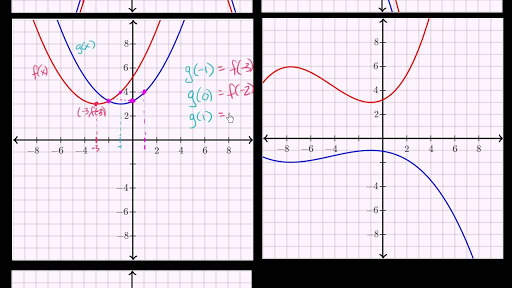
Graph Transformations A transformation is something that is done to a graph/function that causes it to change in some way This topic is about the effects that changing a function has on its graph There are two types of transformation translations and reflections, giving 4 key skills you must be familiar with Throughout this topic, we will use the notation f(x) to refer to a functionThat is, the rule for this transformation is –f (x) To see how this works, take a look at the graph of h(x) = x 2 2x – 3So this red curve is the graph of f of X and this blue curve is the graph of G of X and I want to try to express G of X in terms of f of X and so let's see how they're related so we pick any X and we could start right here at the vertex of f of X and we see that at least at that point G of X is exactly one higher than that so G of two I could write this down G of two G of two is equal to is
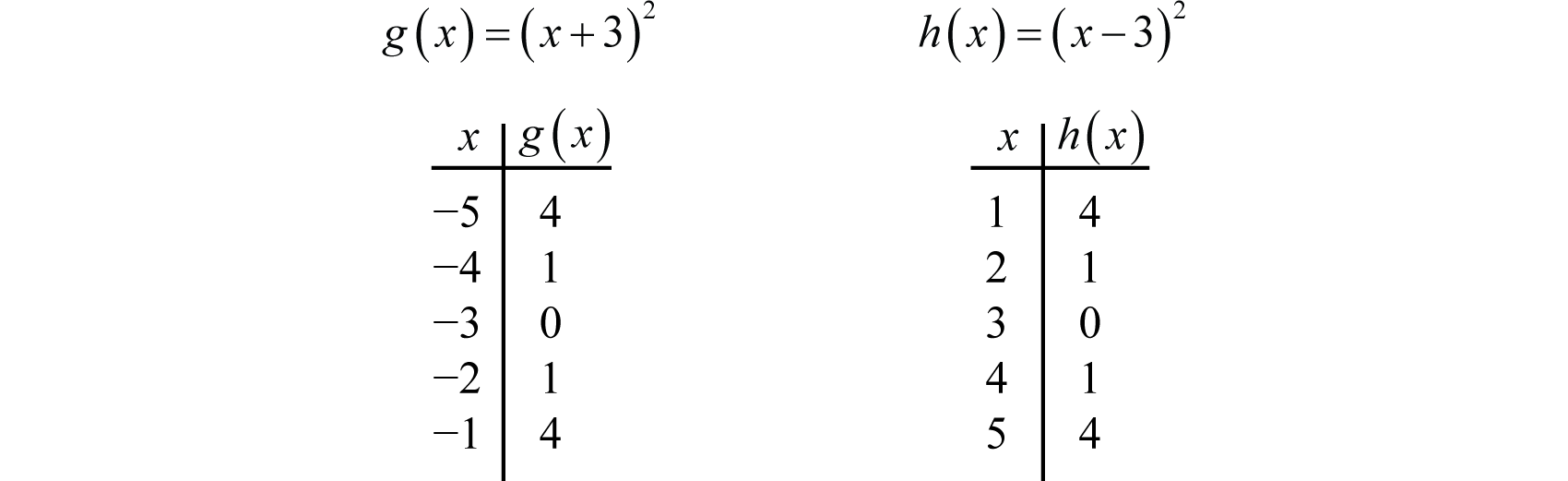



Using Transformations To Graph Functions
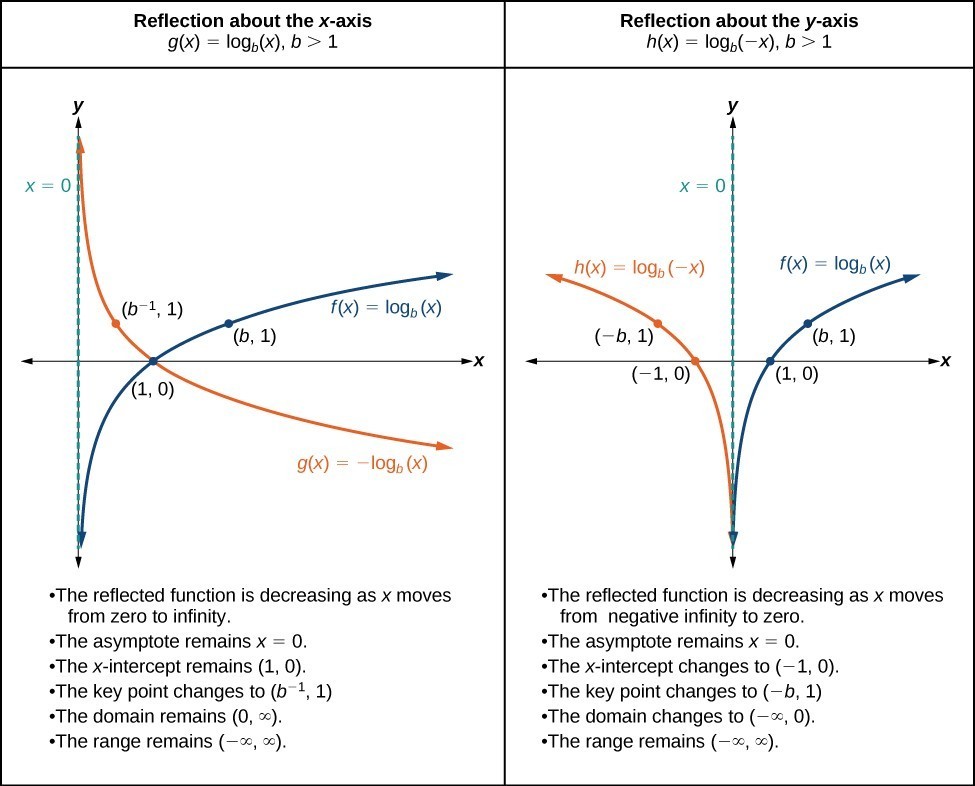



Graphing Transformations Of Logarithmic Functions College Algebra
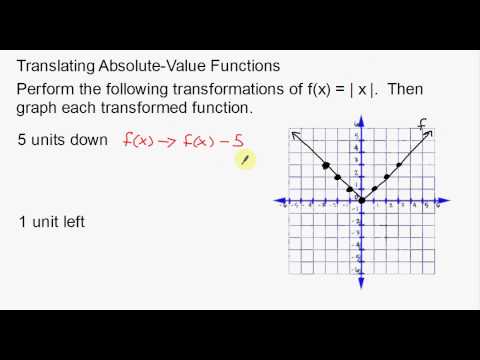
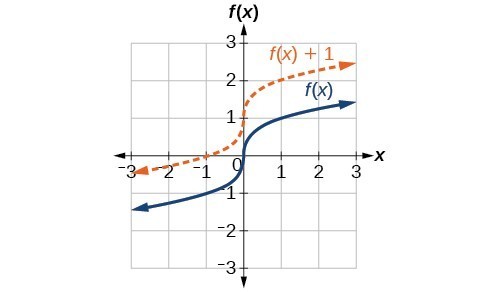
The graph of y = f (x) is the graph of y = f (x) reflected about the yaxis Here is a picture of the graph of g(x) =(05x)31 It is obtained from the graph of f(x) = 05x31 by reflecting it in the yaxis Summary of Transformations To graph Draw the graph of f and Changes in the equation of y = f(x) Vertical Shifts y = f (x) c Graph the basic graph By determining the basic function, you can graph the basic graph The basic graph is exactly what it sounds like, the graph of the basic function The basic graph can be looked at as the foundation for graphing the actual function The basic graph will be used to develop a sketch of the function with its transformationsOn each grid, y = f(x) is drawn Sketch the graph of the transformation indicated Transformations y = f(x) 5 y = f(x) y = f(x) y = f(x 3) 4




How To Graph Transformations Of Functions 14 Steps



Identifying Function Transformations Video Khan Academy
The video explains how to graph the absolute value of f(x) from the graph of f(x)http//mathispower4ucomMultiplying a function by a negative constant, − f (x), reflects its graph in the xaxis Multiplying the values in the domain by −1 before applying the function, f (− x), reflects the graph about the yaxis When applying multiple transformations, apply reflections firstF ( x) = x2 A function transformation takes whatever is the basic function f (x) and then "transforms" it (or "translates" it), which is a fancy way of saying that you change the formula a bit and thereby move the graph around For instance, the graph for y = x2 3 looks like this This is three units higher than the basic quadratic, f (x) = x2
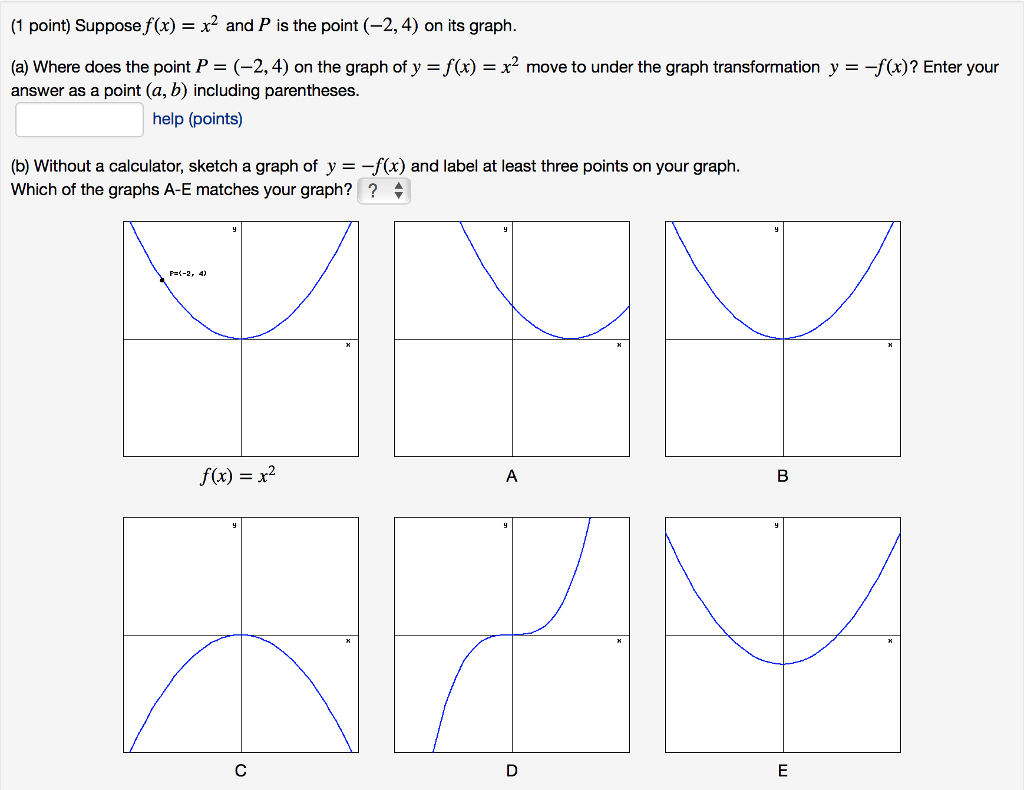



Suppose F X X 2 And P Is The Point 2 4 On Its Chegg Com




Function Transformations
I am a graduate student teaching college algebra at a larger state school, and currently I'm covering transformations of graphs of function, ieGraph transformations Given the graph of a common function, (such as a simple polynomial, quadratic or trig function) you should be able to draw the graph of its related function The 1/x Function f(x) = 1/x looks like it ought to be a simple function, but its graph is a little bit complicated It's really not as bad as it looks, though!




Transforming Graphs Of Functions Brilliant Math Science Wiki



What Is A Function Transformation Expii
Begin by evaluating for some values of the independent variable x Figure 251 Now plot the points and compare the graphs of the functions g and h to the basic graph of f(x) = x2, which is shown using a dashed grey curve below Figure 252 The function g shifts the basic graph down 3 units and the function h shifts the basic graph up 3 unitsWe're talking about graphing transformations Let's start with an easy transformation y equals a times f of x plus k Here's an example y equals negative one half times the absolute value of x plus 3 Now first, you and I ide identify what parent graph is being transformed and here it's the function f of x equals the absolute value of xThis type of graphing transformation can be written as y = f (x h) For this graphing transformation, we shift the graph horizontally by h units We should also know how to recognize vertical shifts and scaling, reflections, and horizontal compression functions transformations horizontal shift end point
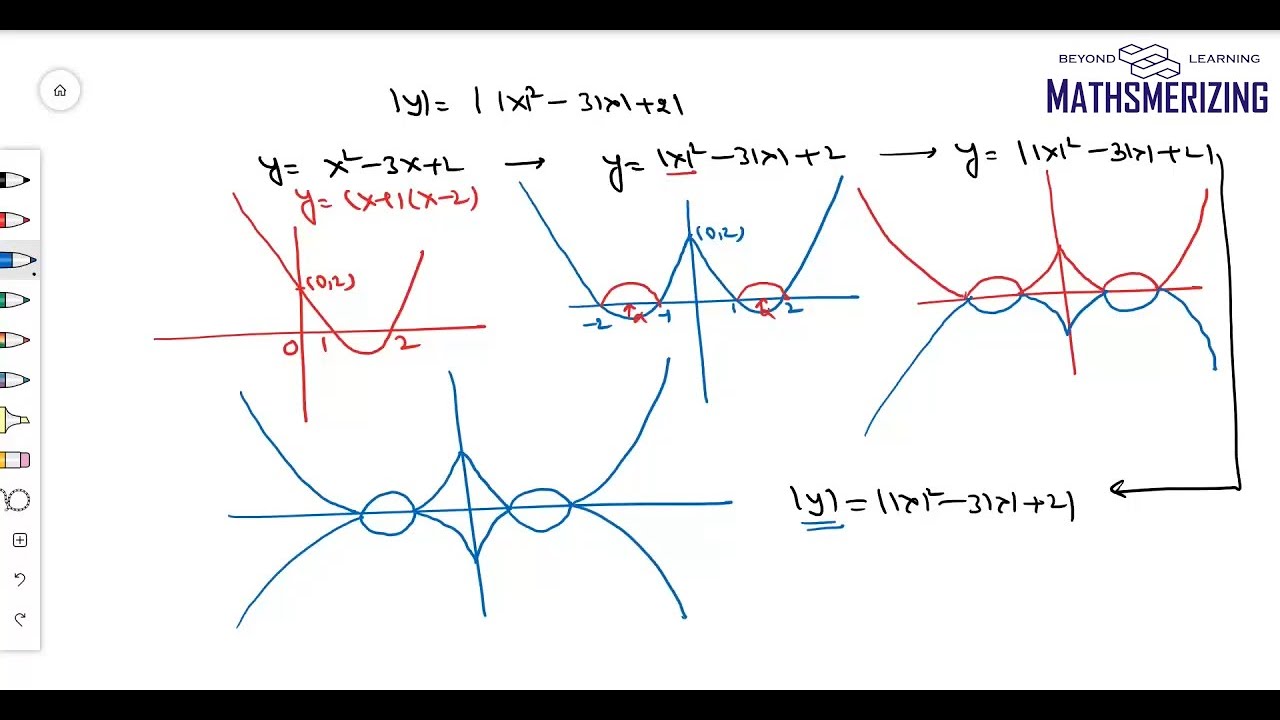



Transformation Of Graph L2 Modulus Function Graphs Of Y F X Y F X Y F X With Examples Youtube
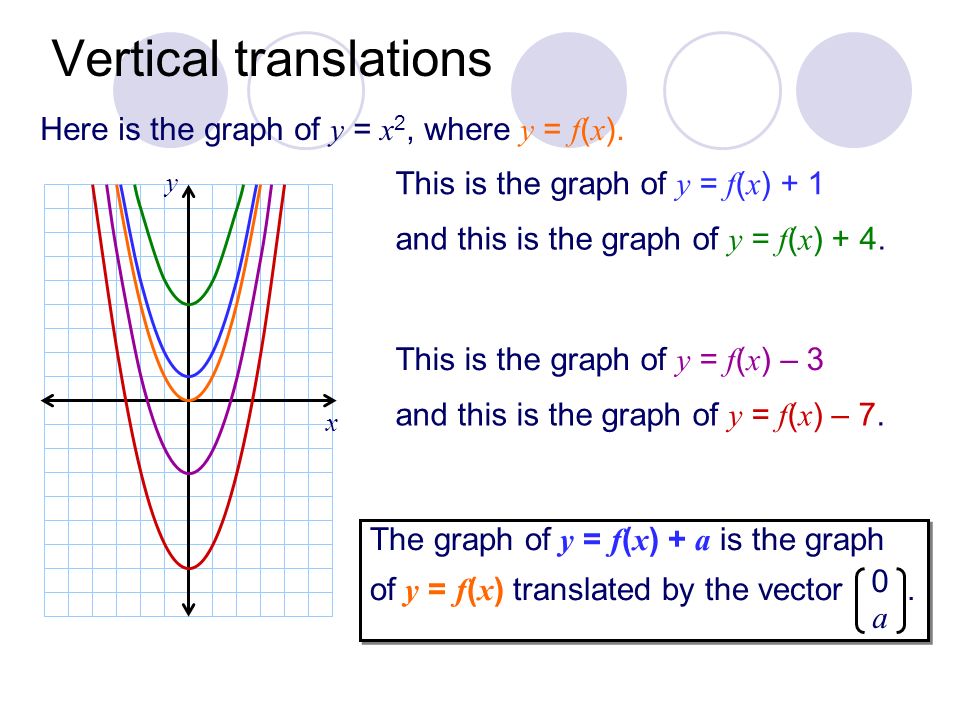



Transforming Graphs Of Functions Ppt Download
Find the value of k given the graphs Experiment with cases and illustrateMr Jeffery demonstrating an easy trick that will allow you to work out graph transformation questions using a simple to follow methodTransformations of Graphs Suppose we know what the graph of y f x looks like If c 0, then we can determine the graph that results when we define some new functions in terms of c, x, and f x Type of Transformation To Graph Do this to the graph of y f x



1
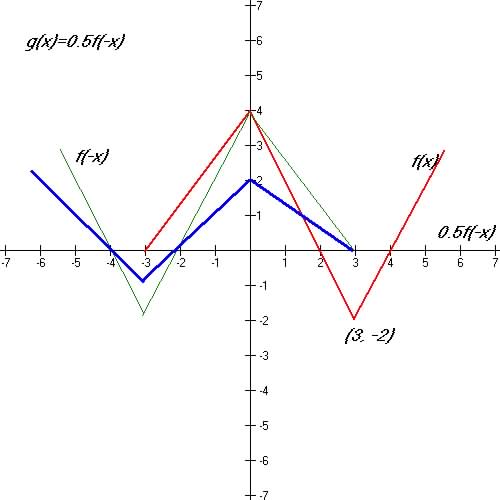



F X Graph Transformation រ បភ ពប ល ក Images
Graph Transformations There are many times when you'll know very well what the graph of a particular function looks like, and you'll want to know what the graph of a • Beginning with the graph f(x)=x2, we can use the chart on the previous page to draw the graphs of f(x 2),f(x 2), f(2x), f(1 2x), and f(x)Purplemath The last two easy transformations involve flipping functions upside down (flipping them around the xaxis), and mirroring them in the yaxis The first, flipping upside down, is found by taking the negative of the original function;Math AA HL Graph Transformations #MAAHL Maximum Mark 6 There exists a graph f (x) for a) Sketch the reflection of this graph over, first, the y axis, and then the x axis b) There exists a graph which can be expressed as Find the value of and Detailed Answers/Video Explanations Answer #MAAHL Maximum Mark 6 Let and



Sat Function Transformations The Definitive Guide The College Panda



Transformation Of Function
To find the transformation, compare the two functions and check to see if there is a horizontal or vertical shift, reflection about the xaxis, and if there is a vertical stretch Parent Function f (x) = x f ( x) = x Horizontal Shift None Vertical Shift Down 4 4 Units Reflection about the xFunctions & Graphing Calculator \square!F x x g x x h x x y o o (c) Yes, parts (a) and (b) yield the same function Example Problem 4 Start with the function f x x , and write the function which results from the given transformations Then decide if the results from parts (a) and (b) are equivalent (a)



What Is A Function Transformation Expii



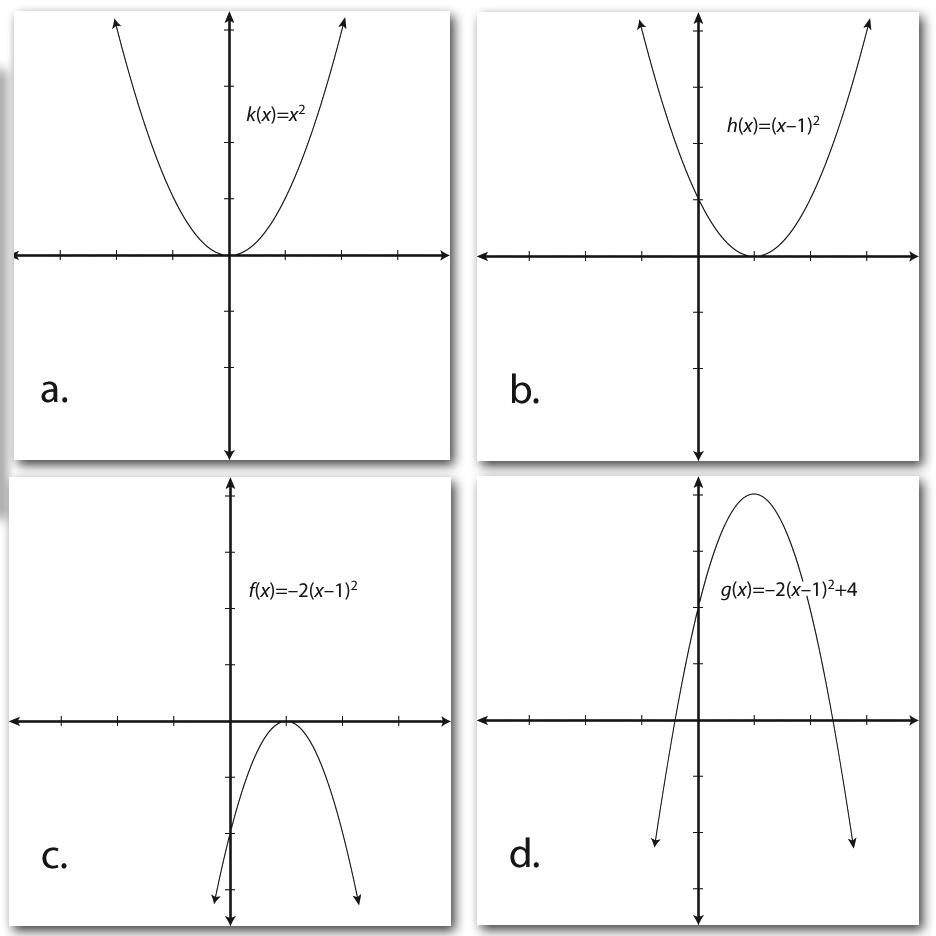
The Graph Of The F X Is Show Below Graph Each Transformed Function And List In Words The Transformation Used Socratic
Graphing Transformations of Logarithmic Functions As we mentioned in the beginning of the section, transformations of logarithmic graphs behave similarly to those of other parent functions We can shift, stretch, compress, and reflect the parent function y = l o g b ( x) \displaystyle y= {\mathrm {log}}_ {b}\left (x\right) y = logExample 960 Graph f(x) = x2 6x 5 by using transformations Solution Step 1 Rewrite the function in f(x) = a(x − h)2 k vertex form by completing the square Separate the x terms from the constant Take half of 6 and then square it to complete the square (1 2 6)2 = 9 Begin by graphing f ( x) = 2 x Then use transformations of this graph to graph the given function Be sure to graph and give equations of the asymptotes Use the graphs to determine each function's domain and range If applicable, use a graphing utility to confirm your handdrawn graphs h ( x) = 2 x 1 − 1 Your answer




Graph Exponential Functions Using Transformations College Algebra



Transformation Of Functions Algebra And Trigonometry
How the x or y coordinates is affected?



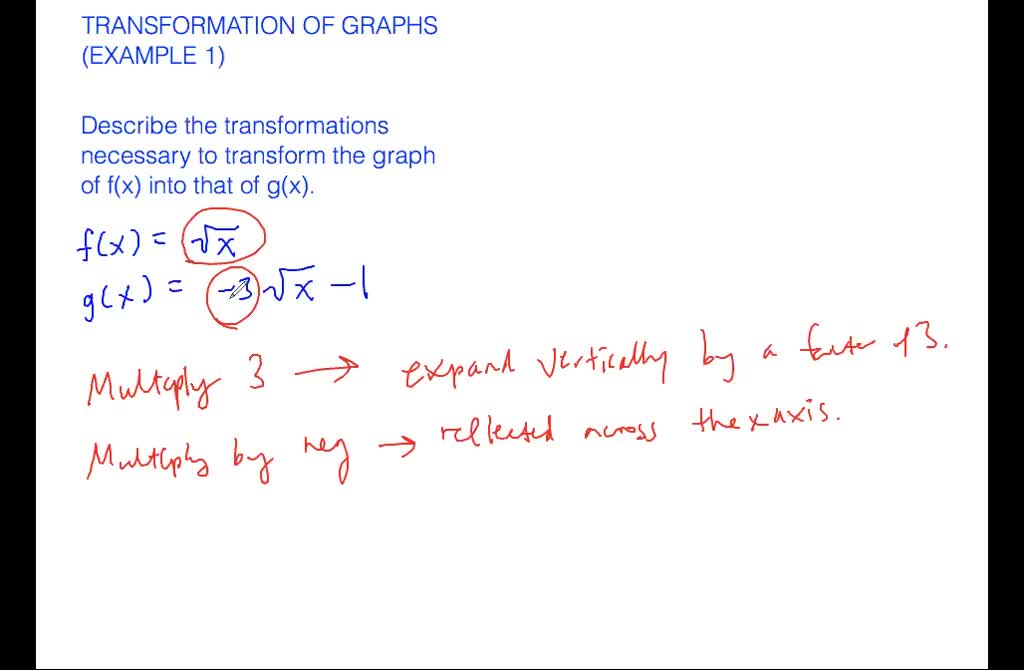
Function Transformations



Consider The Graph Below 10 8 6 4 F X 10 8 6 4 2 Chegg Com
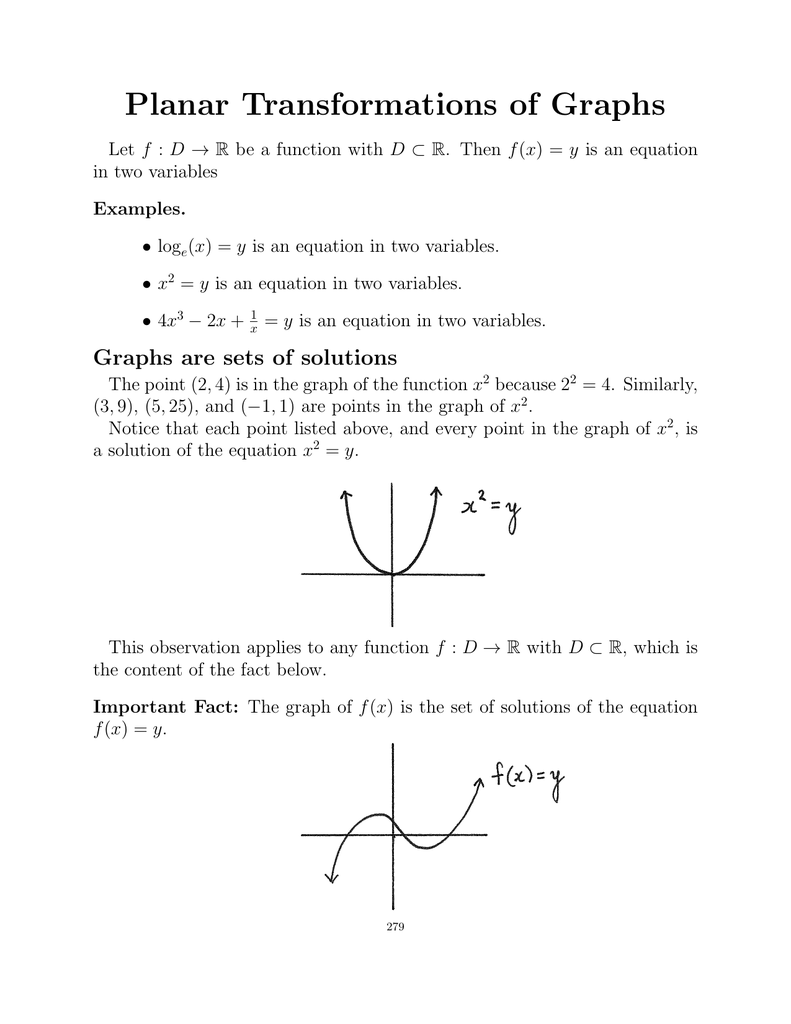



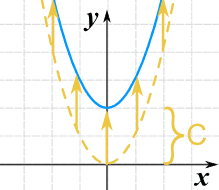
Planar Transformations Of Graphs F X F




Ppt Graph Transformations Powerpoint Presentation Free Download Id




Using Transformations To Graph Functions



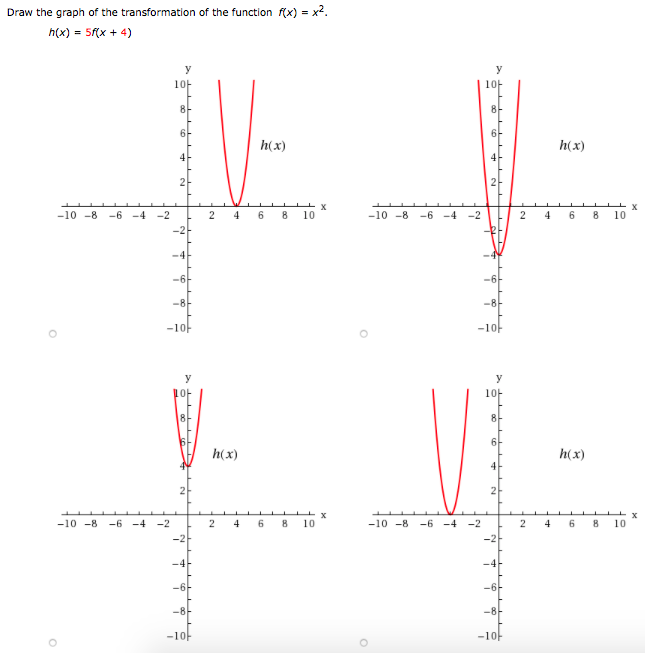
Transformations Of Graphs Example 1 Numerade




The Following Graphs Are Transformations Of F X X 2 Complete The Boxes Below To Describe The Brainly Com




Transformations How To Shift Graphs On A Plane Video Lesson Transcript Study Com
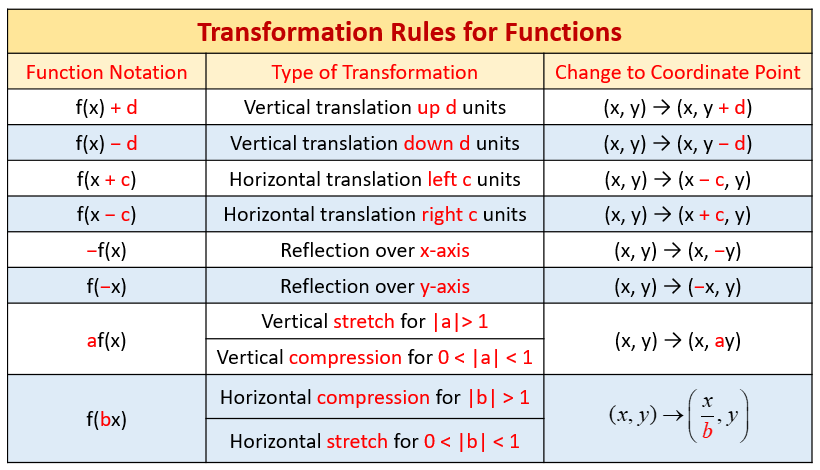



Function Transformation Video Lessons Examples And Solutions
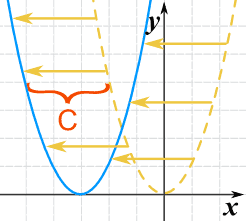



Function Transformations




Calculus Transformations Of Functions Math Open Reference
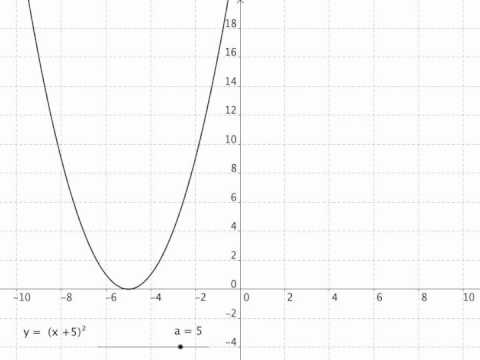



Graph Transformation Y F X A Of The Function F X Youtube
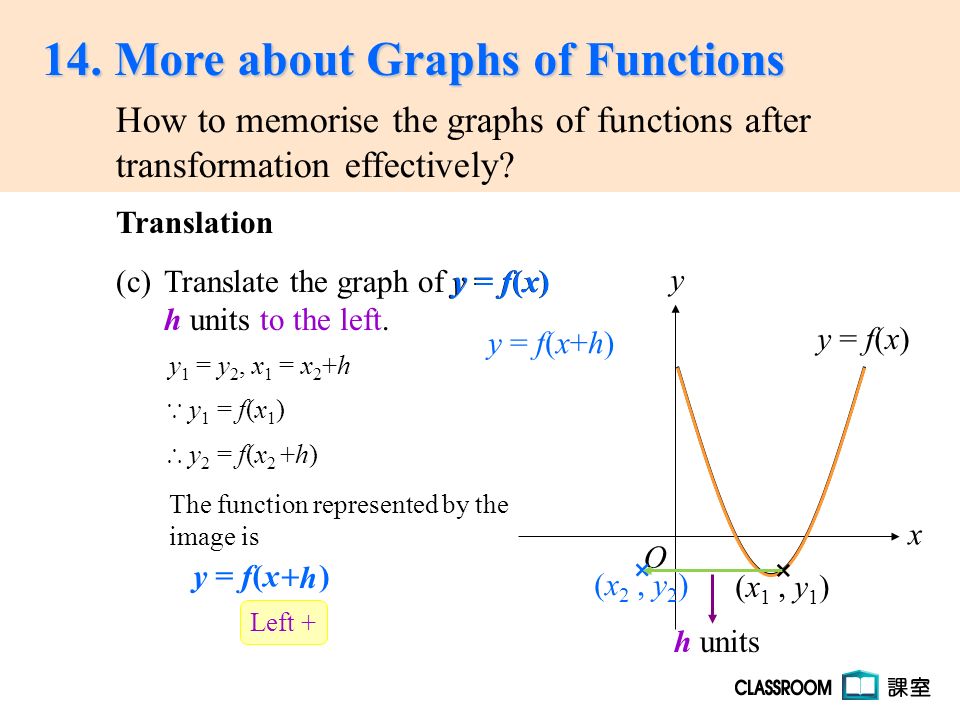



14 More About Graphs Of Functions Transformation Effectively How To Memorise The Graphs Of Functions After O Y X A Translate The Graph Of Y F X K Ppt Download



Transformations Of Functions Mathbitsnotebook A1 Ccss Math
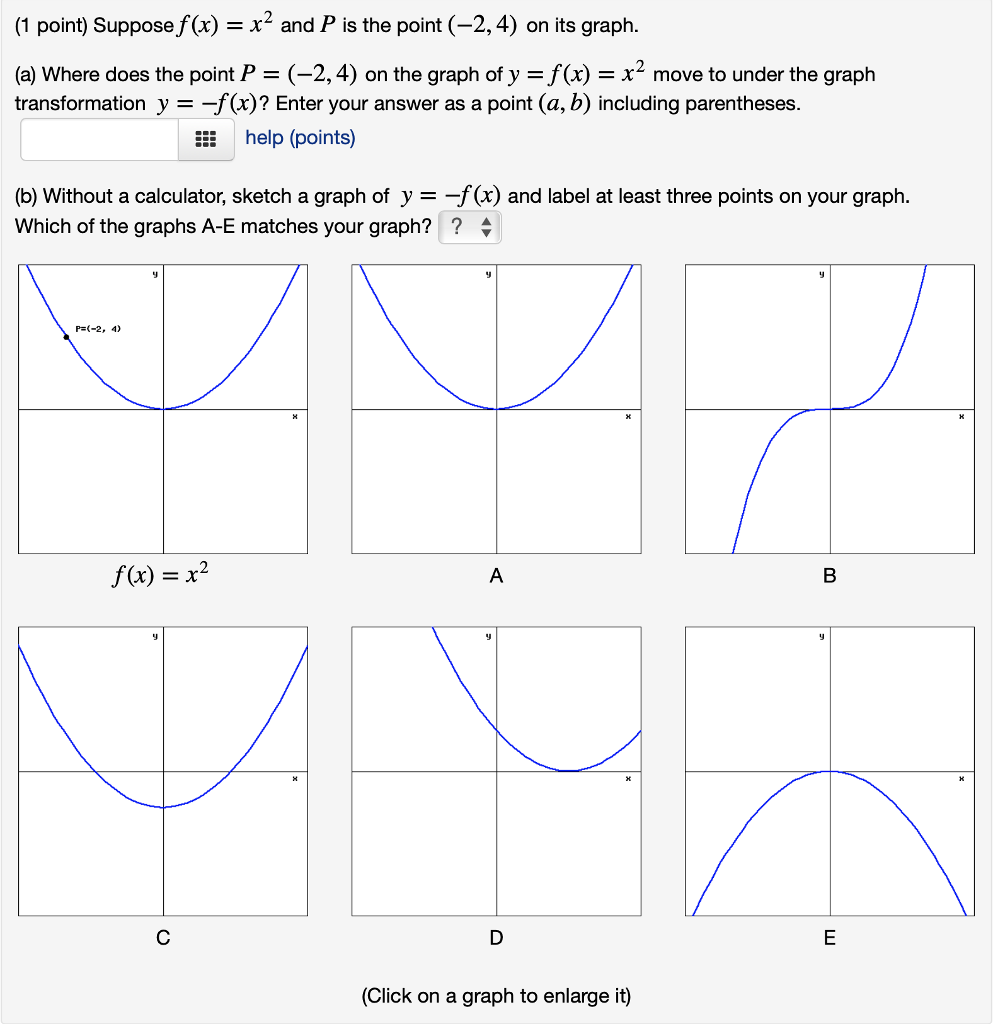



1 Point Suppose F X X2 And P Is The Point 2 4 Chegg Com




How To Graph Transformations Of Functions 14 Steps
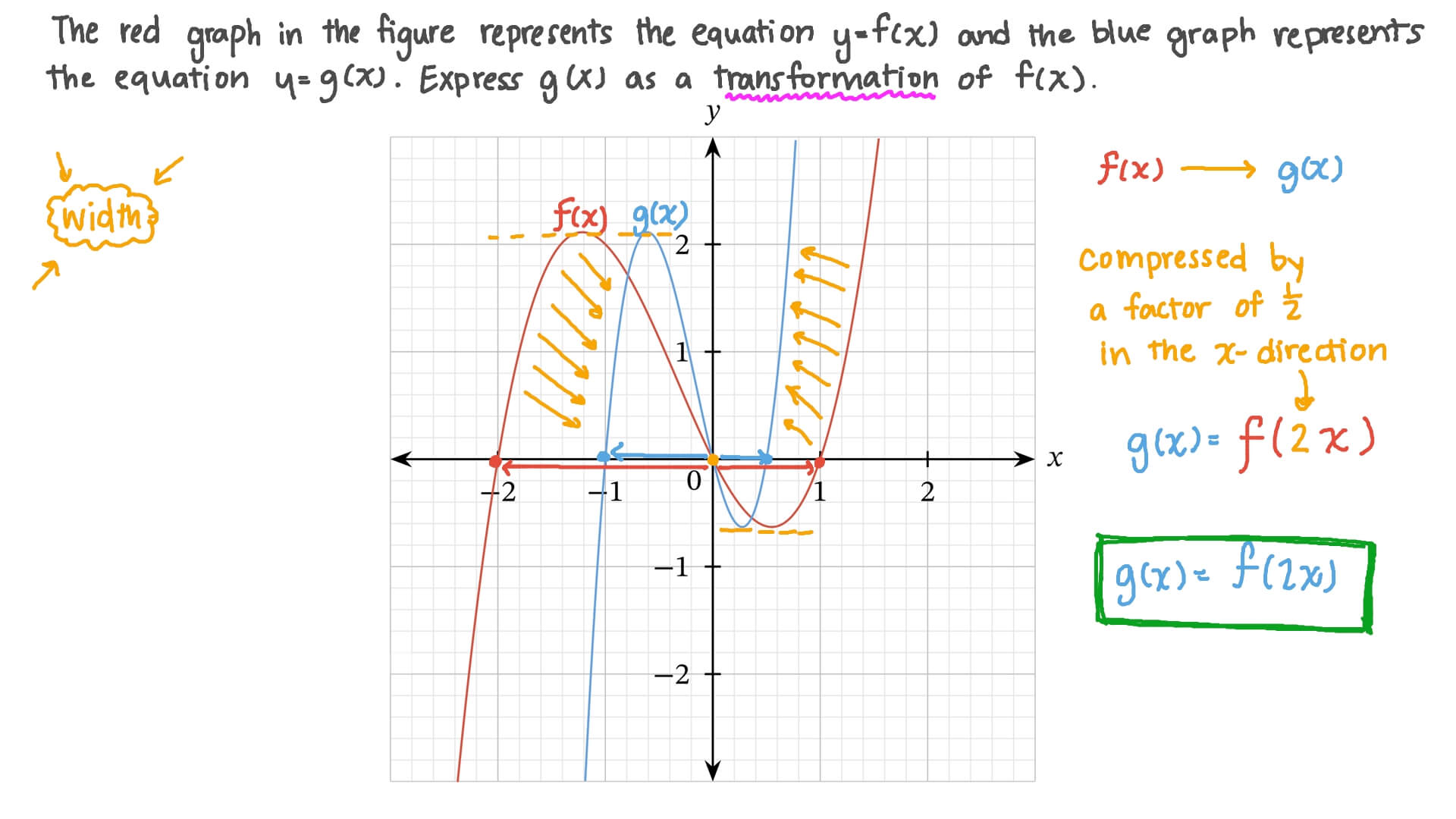



Question Video Transformations Of Graphs Nagwa




Simple Graph Transformation Question Rightarrow 1 F X Mathematics Stack Exchange
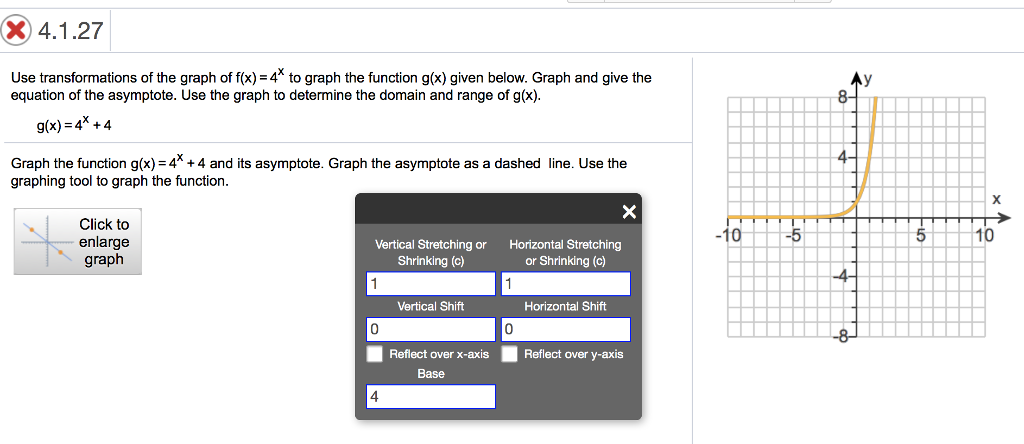



Use Transformations Of The Graph Of F X 4 X To Chegg Com
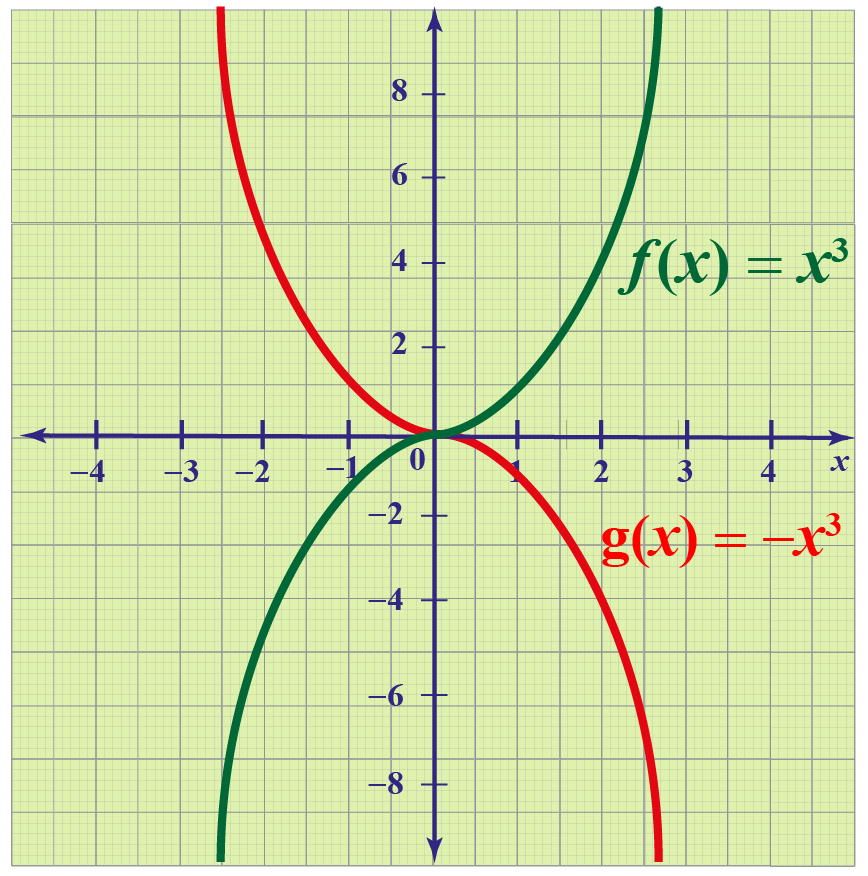



Transformations Of Functions Definitions Facts And Solved Examples Cuemath



Transformations Of Functions College Algebra




Transformations Of Graphs Reflections Y F X Y F X Proof Examsolutions Youtube



Graph Transformations 2 Fx A Copy Youtube



Q Tbn And9gcsl0jyluxfxjjcizs7jyiyflsxbm6hpbpzdawckl4kjhmo0pmir Usqp Cau




Graph Transformations With Examples Gcse Mitch Maths



Biomath Transformation Of Graphs



Transformations Of Functions Mathbitsnotebook A1 Ccss Math



Biomath Transformation Of Graphs



Algebra Graph Transformations Examsolutions



1



Function Transformations



Biomath Transformation Of Graphs




Transformations Of Functions Mathematics Learning And Technology
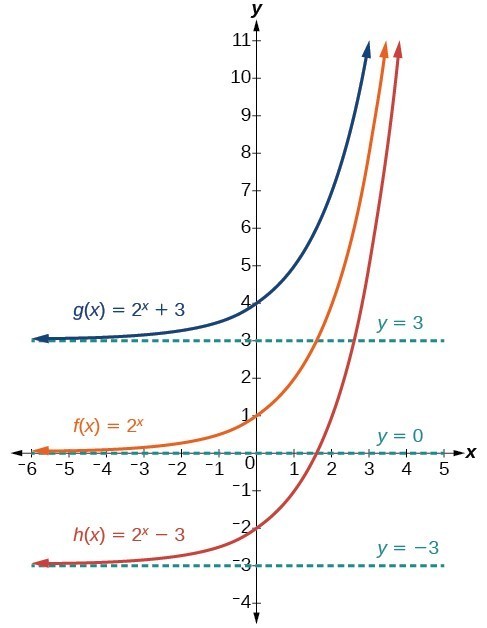



Graph Exponential Functions Using Transformations College Algebra




Graph The Result Of The Following Transformation On F X Brainly Com
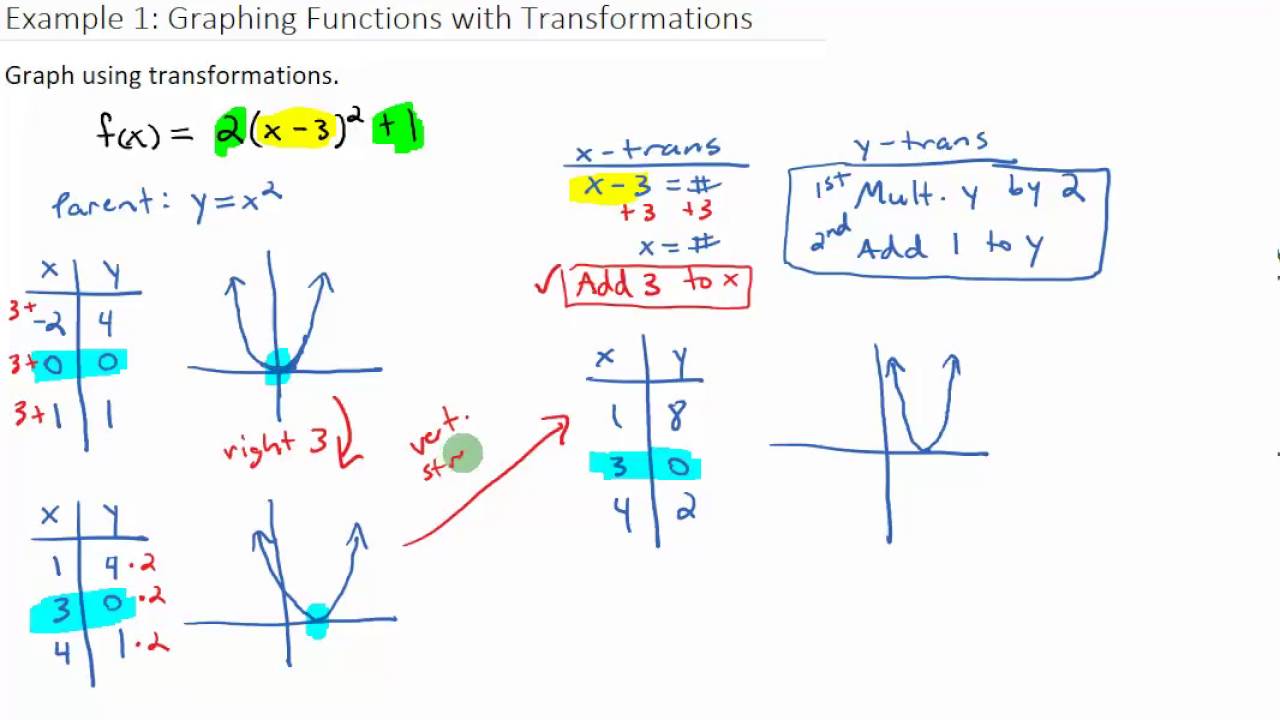



Example 1 Graphing A Function With Transformations Youtube
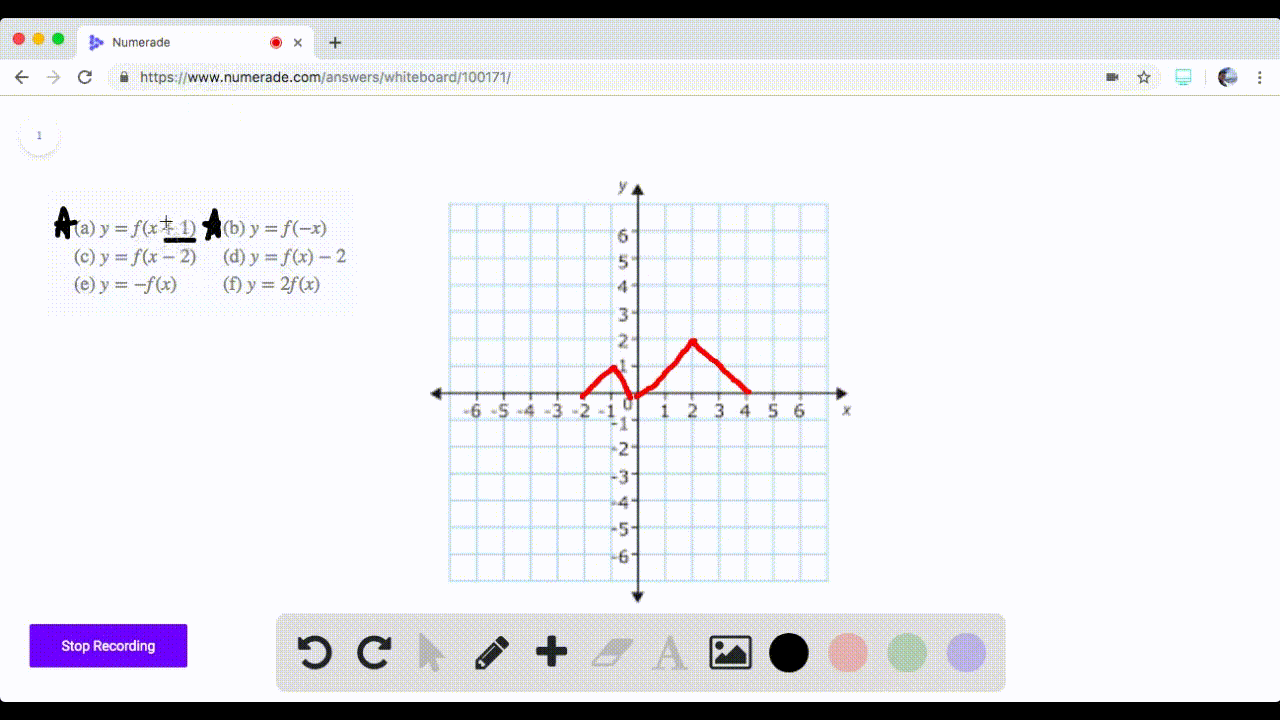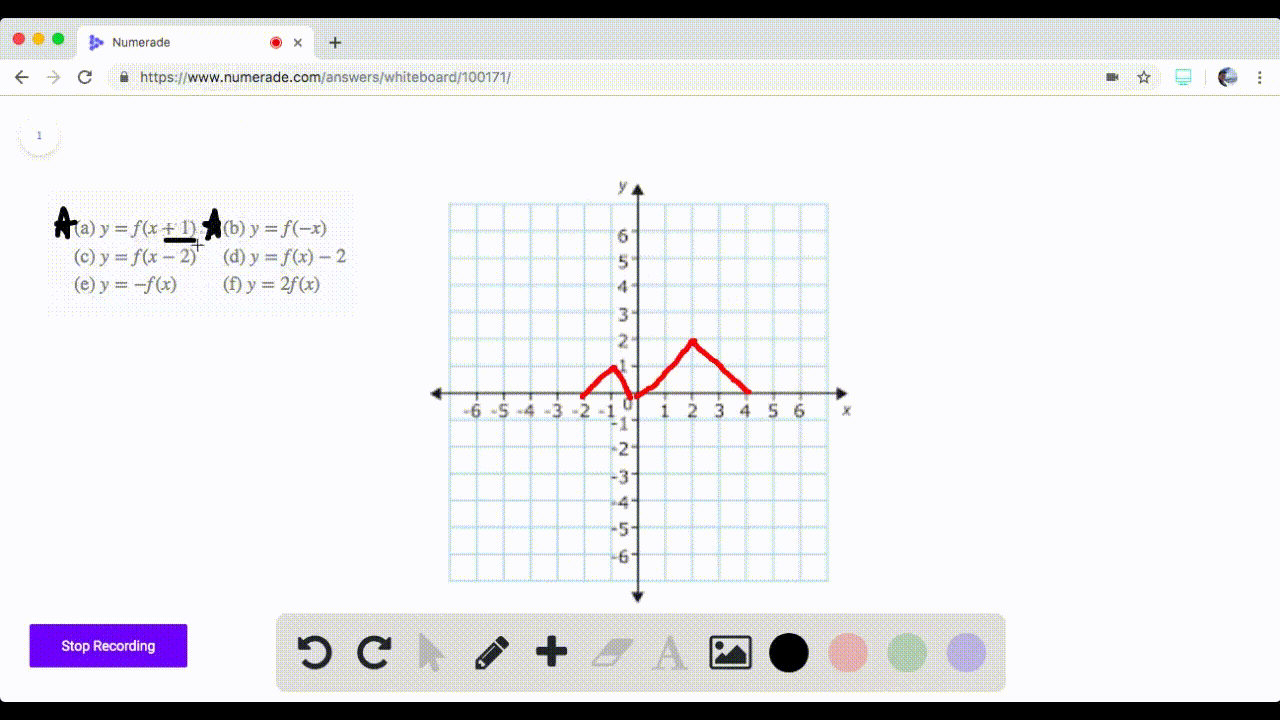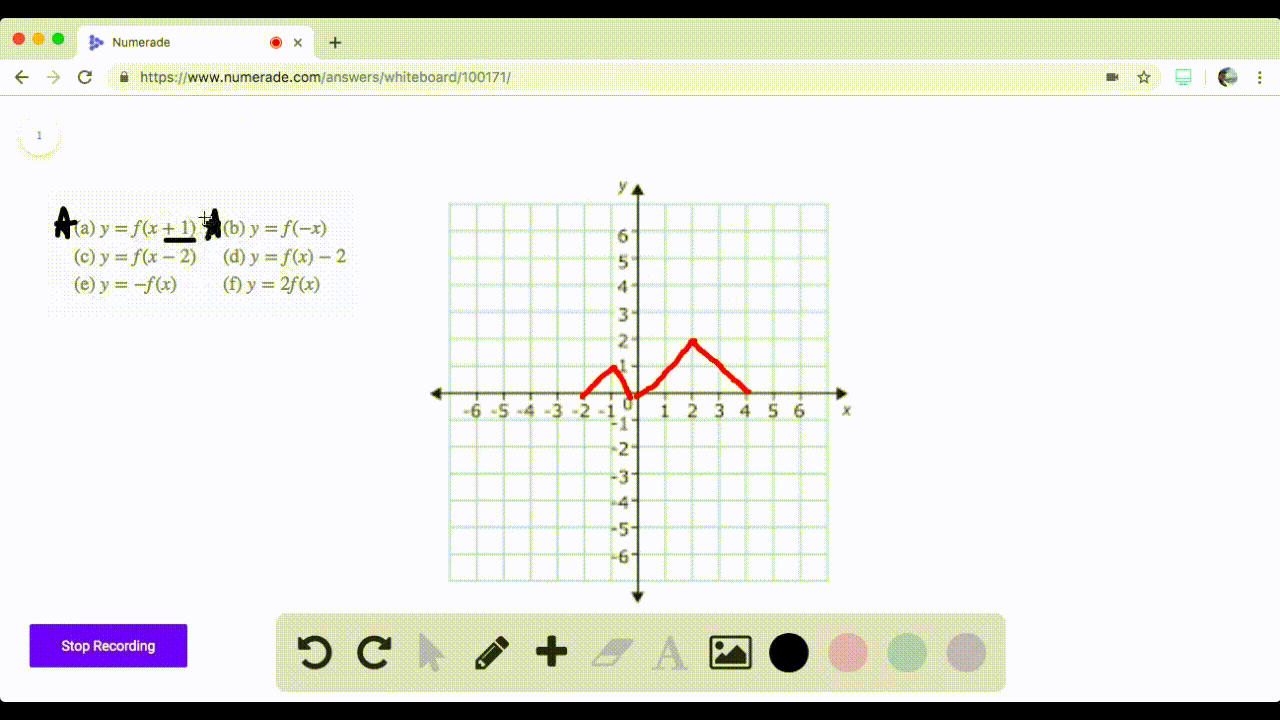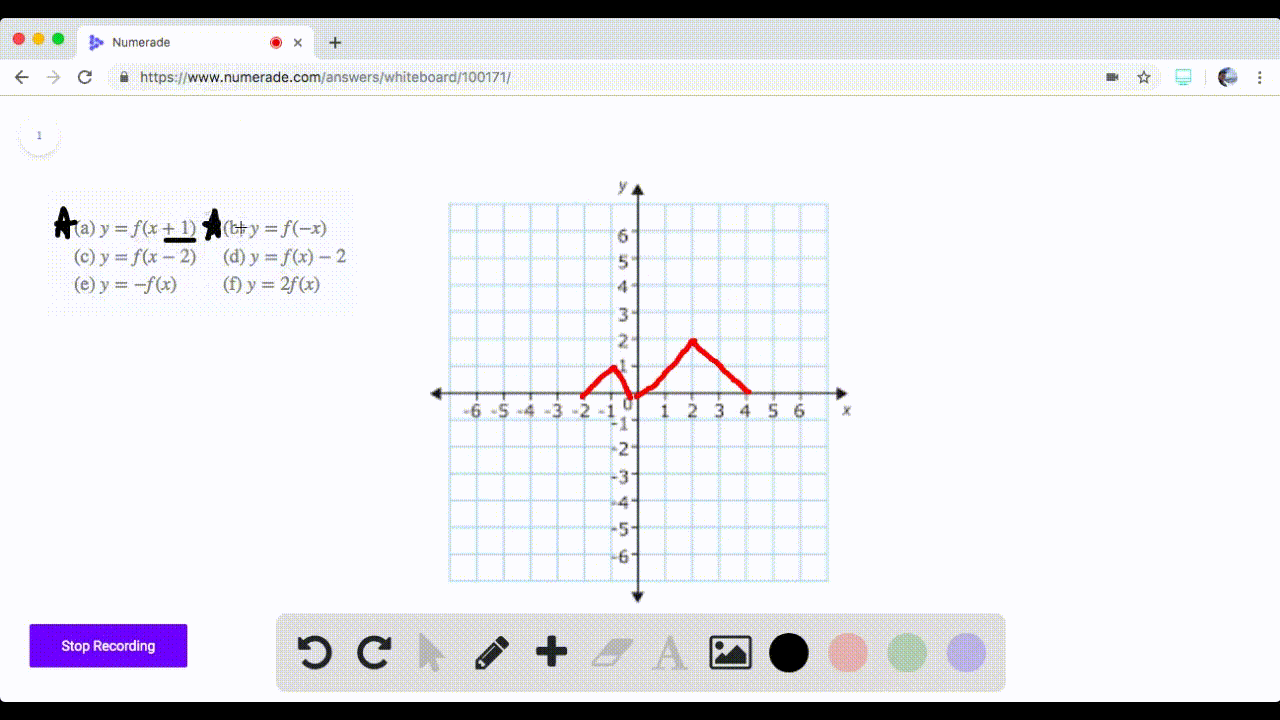



Solved 71 74 Graphing Transformations The Graph Of A Function F Is Given Sketch The Graphs Of The Following Transform
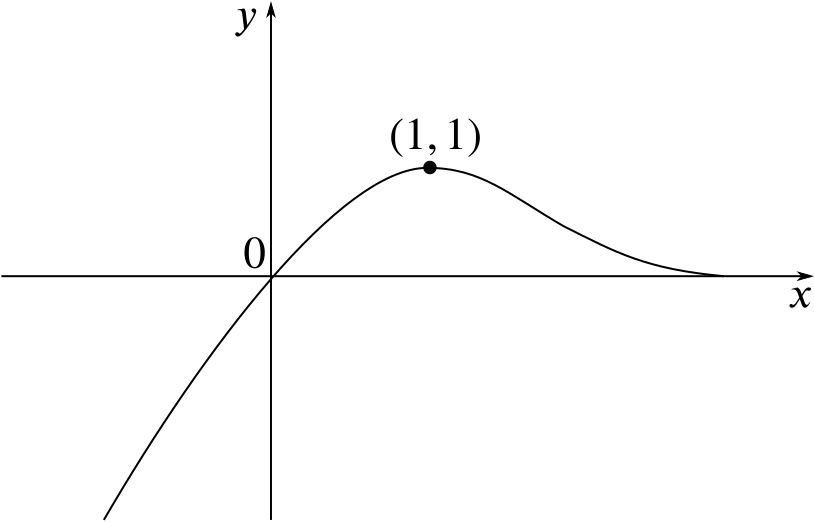



Solution How Do These Transformations Change The Graph Of F X Combining Functions Underground Mathematics



Transformations Of Functions Mathbitsnotebook A1 Ccss Math
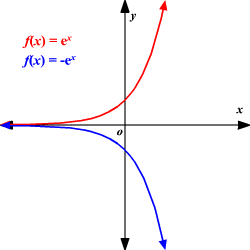



Transformation Of Function



1 5 Shifting Reflecting And Stretching Graphs




Reflecting Compressing Functions Video Khan Academy




Precalculus Shifting Reflecting And Stretching Graphs Flashcards Quizlet
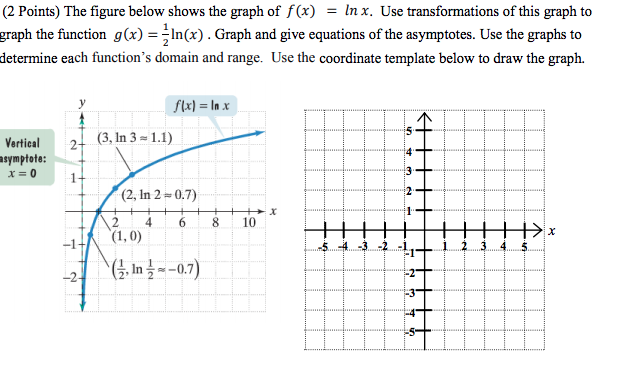



2 Points The Figure Below Shows The Graph Of F X Chegg Com




Transformations Of Functions Ck 12 Foundation




Stretching And Reflecting Transformations Read Algebra Ck 12 Foundation
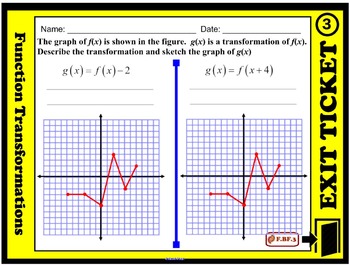



Exit Ticket Function Transformations Random Graph Of F X Tpt
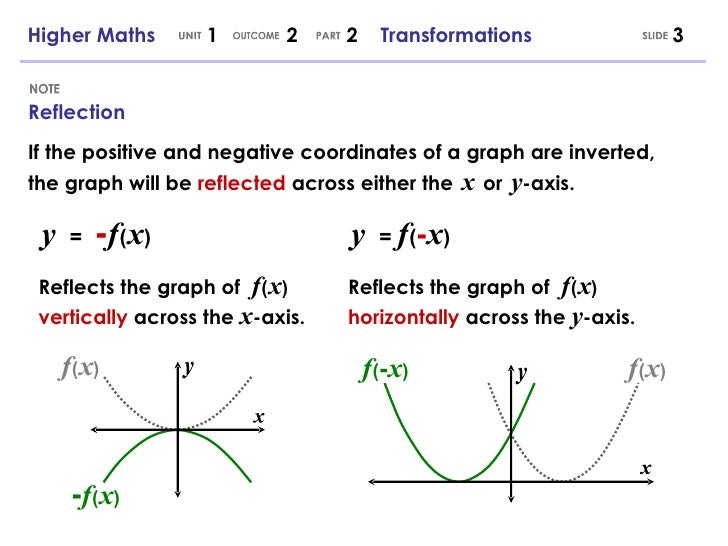



Higher Maths 1 2 2 Graphs And Transformations
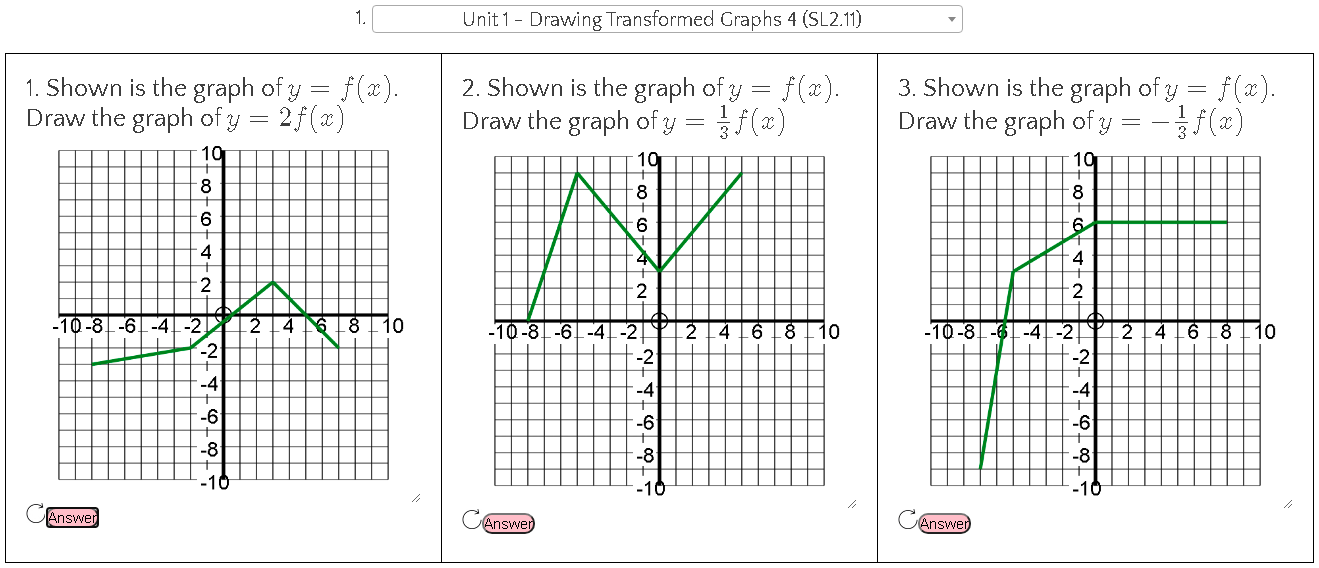



Graph Transformations In Zoom




Simple Graph Transformation Question Rightarrow 1 F X Mathematics Stack Exchange




Combining Transformations Read Algebra Ck 12 Foundation
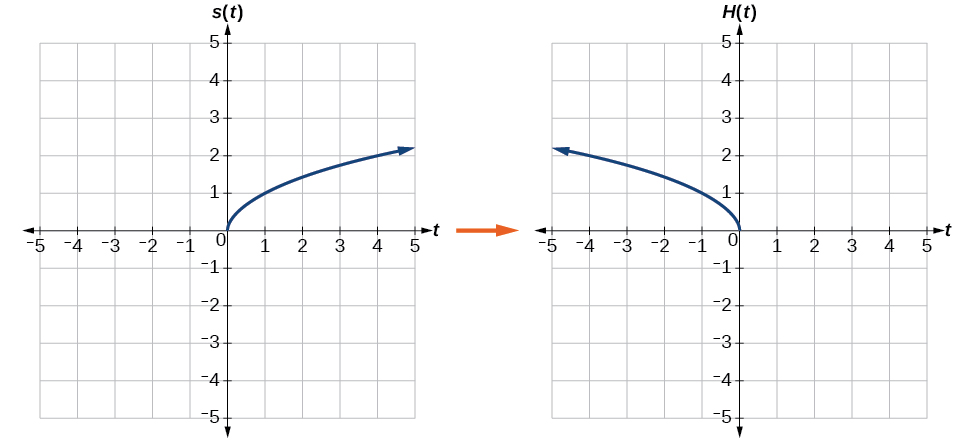



Transformation Of Functions Algebra And Trigonometry
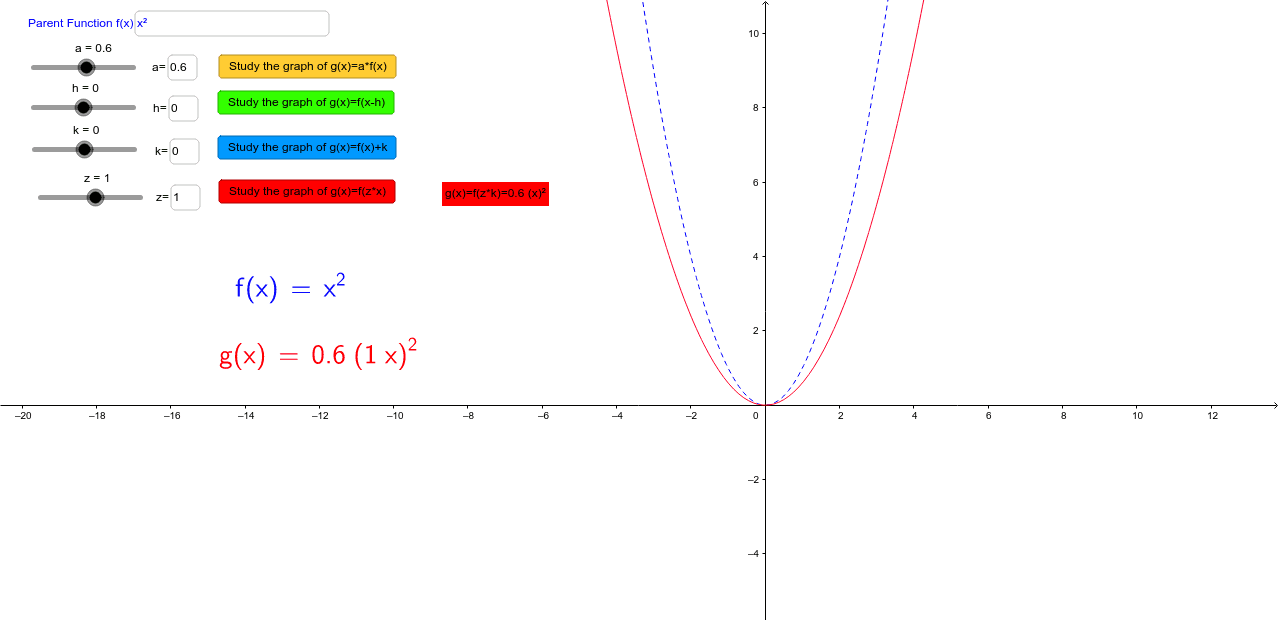



Graph Transformations Discovering Manipulating Functions Geogebra




Transformation Of Function



Transformations Mrs F X
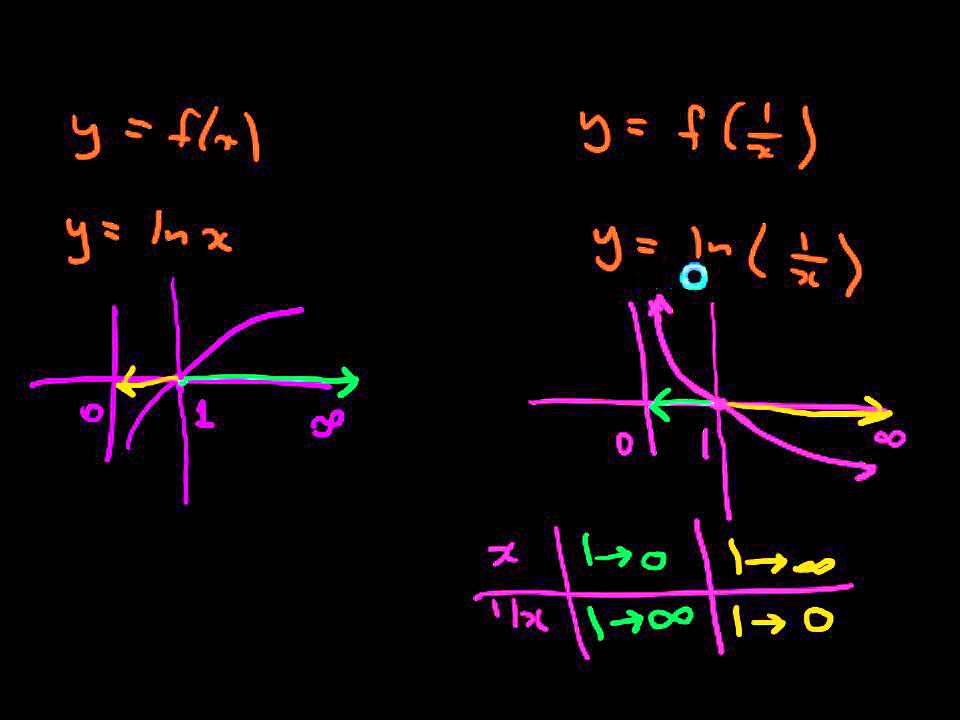



Graphing Transformation Y F 1 X Youtube
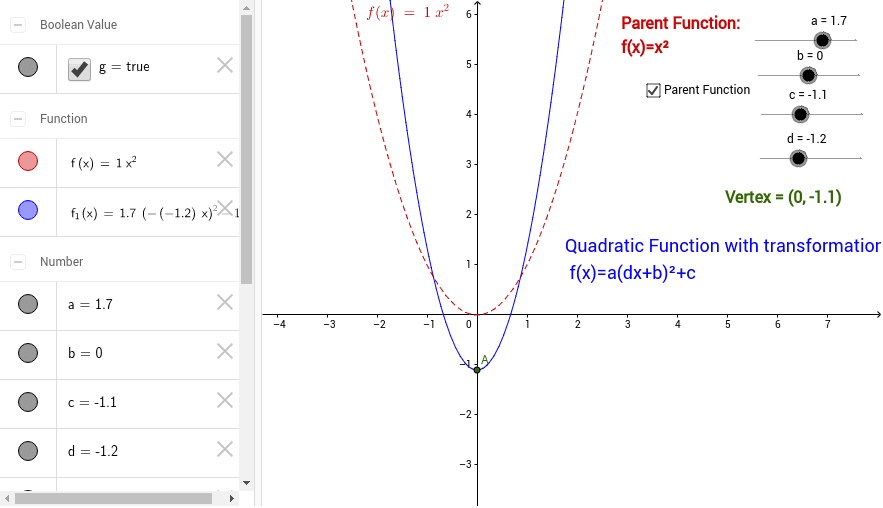



Function Transformations Geogebra




Vertical And Horizontal Transformations Read Algebra Ck 12 Foundation



Biomath Transformation Of Graphs




Using Transformations To Graph Functions



Graphing With Transformations Quantitative Reasoning




Geogebra In The Classroom Single Graph Transformations




Transformations Of The 1 X Function Video Lesson Transcript Study Com
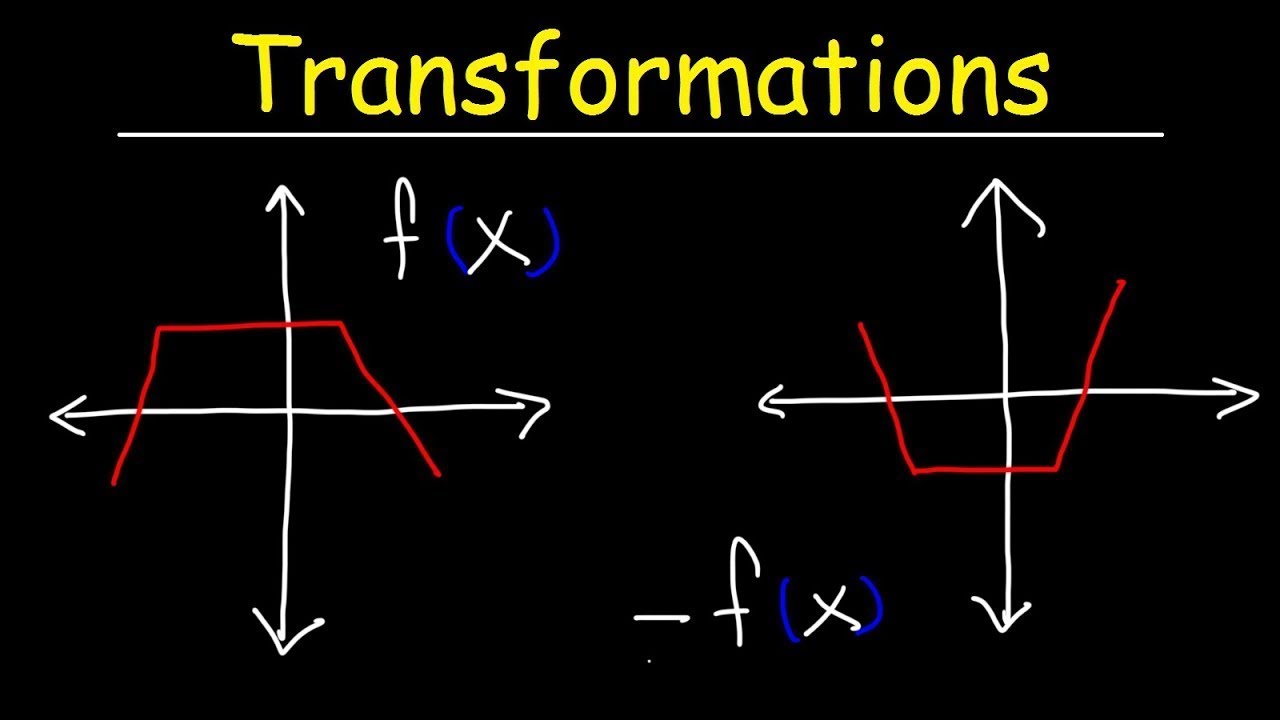



Transformations Of Functions Youtube




Begin By Graphing The Absolute Value Function F X Chegg Com



Transformations Of Functions Mathbitsnotebook A1 Ccss Math



Search Q Unit Circle Tbm Isch
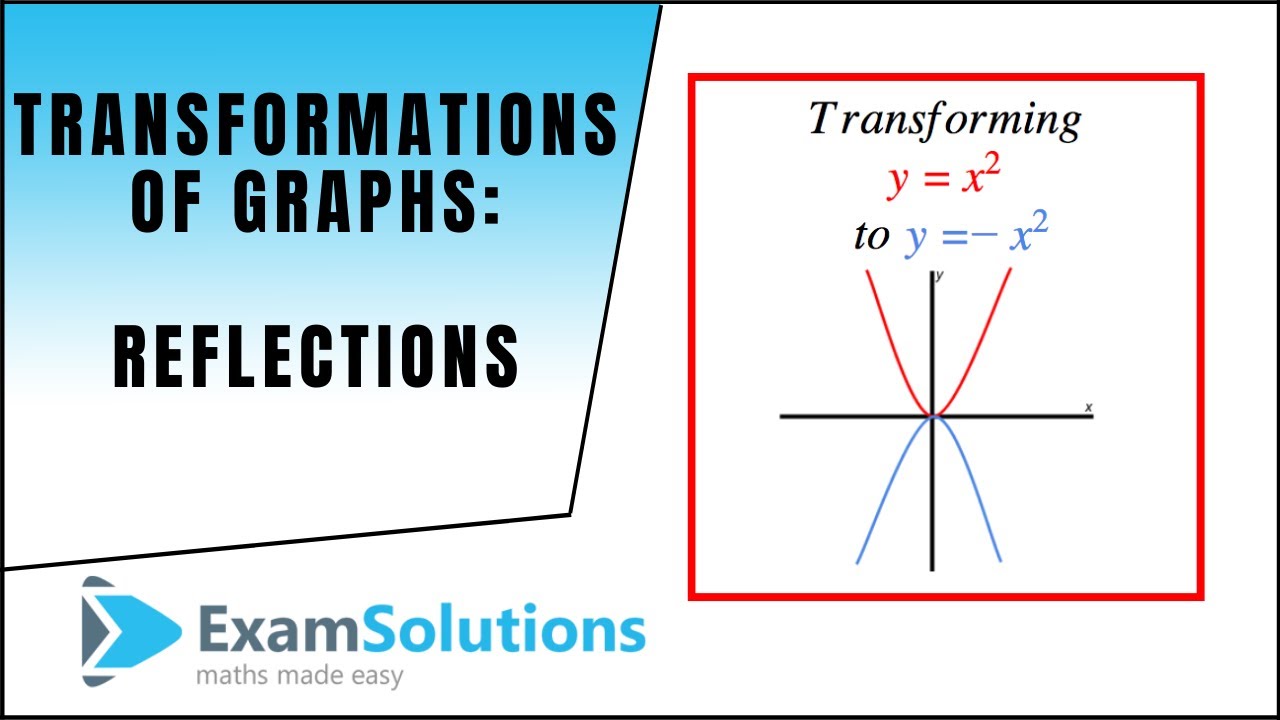



Transformations Of Graphs Reflections Y F X F X Examsolutions Youtube
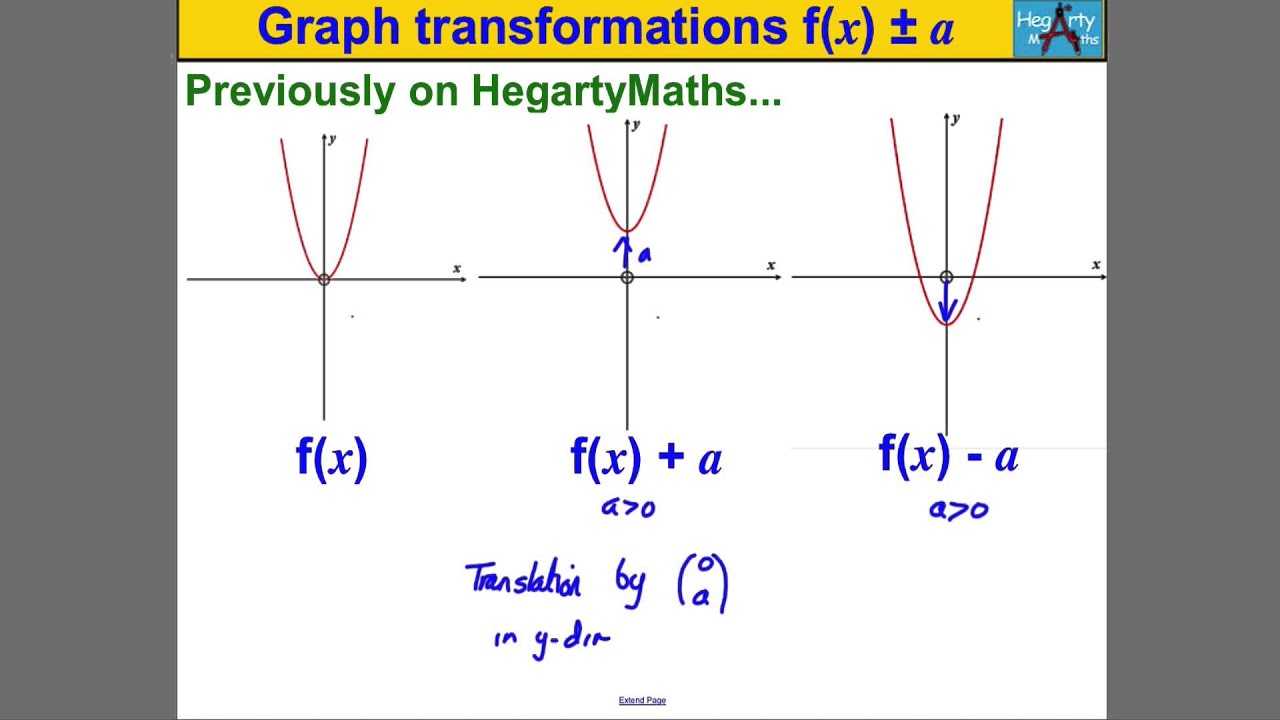



Transforming Graphs Revision Videos Tutor2u



Graphical Transformation Functions F X Wethestudy



Solution Using The Graph Of F X X 2 As A Guide Describe The Transformations And Then Graph Each Function G X 3 X 2 2 This The One Im Havin Problem With Hope Y




Transformation Of Graphs Ppt Video Online Download



Transformations Of Functions Mathbitsnotebook A1 Ccss Math
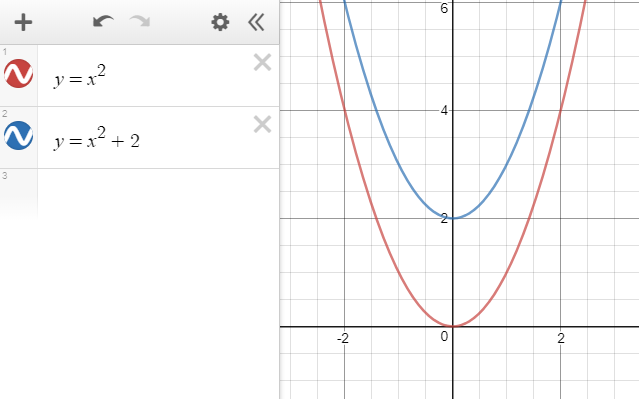



Desmos 2 Transformations Of Graphs Cambridge Maths Hub




The Graph Shown Is A Transformation Of The Parent Function F X X Choose The Equation Which Represents The Transformation Shown Study Com



Transformations Of Functions Definitions Facts And Solved Examples Cuemath




Transformations Of The 1 X Function Video Lesson Transcript Study Com
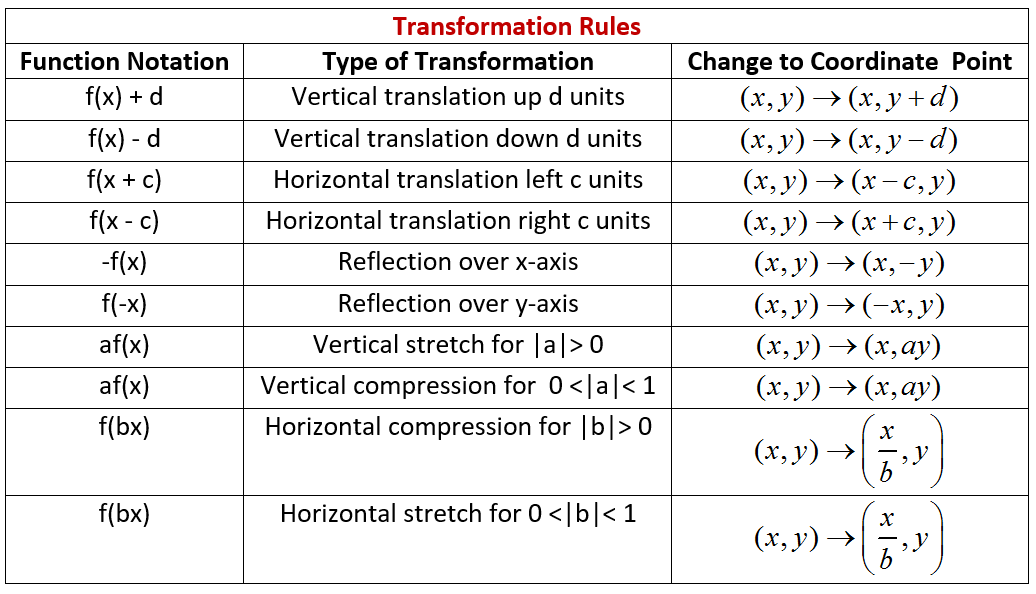



How Do You Sketch The Graph Of Y X 2 2 2 And Describe The Transformation Socratic



No comments:
Post a Comment